Arithmetic Sequence Calculator
Created By : Abhinandan Kumar
Reviewed By : Rajashekhar Valipishetty
Last Updated : Mar 20, 2023
Solving Arithmetic Sequences can be easy from now with this free online tool. Enter the input numbers separated with commas and find the summation of the Arithmetic Sequence in a fraction of seconds. This online Arithmetic Sequence Calculator tool makes the calculations faster & easier.
How to Solve Arithmetic Sequence?
It takes much time to find the highest nth term of a sequence. On a general note, it is sufficient if you add the n-1th term common differences to the first term.
In case all the common differences are positive or negative, the formula that is applicable to find the arithmetic sequence is an = a1+(n-1)d. It is also used for calculating the nth term of a sequence. In case of the zero difference, the numbers are equal and there is no need to do further calculations.
- The process to find the summation of an arithmetic sequence is easy and simple if you follow our steps. They are as below
- To solve the summation of a sequence, you need to add the first and last term of the sequence.
- Later, multiply them with the number of pairs. Mathematically, S = n/2 * (a₁ + a)
- If you substitute the value of arithmetic sequence of the nth term, we obtain S = n/2 * [2a₁ + (n-1)d] after simplification. By using this formula, we can easily find the summation of arithmetic sequences.
For practical understanding of the concept, go with our Arithmetic Sequence Calculator and provide the input list of numbers and make your calculations easier at a faster pace. Also, look at the below solved example and learn how to find arithmetic sequences manually.
Example:
Find the sum of the arithmetic sequence of 2,4,6,8,10,12,14,16?
Solution:
Given series is 2,4,6,8,10,12,14,16
a is the first term and d is the common difference
The general representation of arithmetic series is a, a + d, a + 2d,...a + d(n−1)
n is the total numbers in that series
As per the rule or formula, we can write an Arithmetic Sequence as:
xn = a + d(n−1) (We use "n−1" because d is not used in the 1st term)
(16) = (2) + d(8-1)
7d = (16)-(2)
d = (16)-(2) / 7
d = 2
By using the formula, we can find the summation of the terms of this arithmetic sequence. So, k=1Σn-1 a+kd = n / 2(2a+(n-1)d)
= k=1Σ7 (2)+k × (2) = 8 / 2(2(2)+(8-1)(2)) [put the values in the formula and simplify]
= 8 / 2(2(2)+(7)(2))
= 8 / 2(2(2)+(14))
= 8 / 2((4)+14)
= 8 / 2(18)
= 144 / 2
= 72.0
Hence, the Solution of Arithmetic Sequence of 2,4,6,8,10,12,14,16 is 72.0.
Example for Finding nth term of Arithmetic Sequence
Example for Finding Sum of n terms of Arithmetic Sequence
What is Meant by Arithmetic Sequence or Arithmetic Progression(AP)?
As we all know that the ordered list of numbers is known as a sequence. An arithmetic sequence is one of the types of sequence in math. It is defined as the ordered list of numbers with a certain pattern. In an Arithmetic Sequence, the difference between one term and the next is a constant. The general form of writing an arithmetic sequence is as below:
{a, a+d, a+2d, a+3d, ... }
where,
a is the first term, and
d is the difference between the terms (called the "common difference")
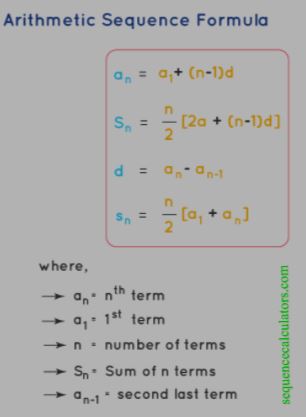
Arithmetic Sequence Formula
The formula to find the arithmetic sequence is given as,
Formula 1: This arithmetic sequence formula is referred to as the nth term formula of an arithmetic progression.
an = a1 + (n-1)d
where,
an = nth term,
a1 = first term, and
d is the common difference
Formula 2: The formula to find the sum of first n terms in an arithmetic sequence is given as,
Sn = n/2[2a + (n-1)d]
where,
Sn = sum of n terms
a1 = first term
d is the common difference between the successive terms
Formula 3: The formula for calculating the common difference of an AP (Arithmetic Progression) is given as,
d= an - an-1
where,
an = nth term,
an-1 = second last term, and
d is the common difference
Formula 4: The formula for summation of first n terms of an arithmetic progression when the first and the last terms are known is,
Sn = n/2[a1 + an]
where,
Sn = Sum of first n terms
an= last term
a1= first term
FAQs on Common Difference Arithmetic Sequence Calculator with Solution
1. What Is Arithmetic Sequence Formula in Algebra?
In algebra, the formula of Arithmetic Sequence is a simple approach to calculate the general term of an arithmetic sequence and the sum of the n terms of an arithmetic sequence.
2. What are the formulas to find the Arithmetic Sequence?
Arithmetic sequence formula calculator, arithmetic sequence solver, arithmetic sequence solver with solution, sum of arithmetic sequence calculator, formula for arithmetic sequence calculator, and nth term calculator can be calculated manually or through tool.
3. How do you find the sum of arithmetic series?
The sum of an arithmetic series is found by multiplying the number of terms times the average of the first and last terms.
4. Where can I find the online Arithmetic Sequence Calculator with Steps?
You can find the free online Arithmetic Sequence Calculator with Steps from our reliable and trusted website ie., SequenceCalculators.com